Making Sense of Division of Fractions
Sometimes it is difficult to make sense of division of fractions problems. Here are four videos that help teachers see how familiar division models fit into situations that use fractions.
Note that this page and these videos are about why we should use division in certain situations, and how to think about it. They are not about how to perform the calculations. For more insight into that issue, see Delaying Invert and Multiply.
"Markers in a Box"
Division of fractions using the measurement model...
This example has no remainder. See the next video for a "remainder" situation.
Why is this kind of division called measurement? You can think of it as measuring the width of the box in "markers." Think back to elementary school where they measure things in paper clips and other non-standard units. This leads to one of the fundamental meanings of division.
Of course, to figure out how many units long something is, you can just lay them out and try it.
But if that's impractical, you can calculate the number of units by dividing the distance by the length of the unit. The context doesn't have to be about distance. Here are a few examples:
- It takes twelve minutes for the crew to wash a car. How many cars can they wash in four hours?
- You can keep a kindergartner happy with one and a half candy bars. You have 20 candy bars. How many kindergartners can you keep happy?
- Madeline can carry 25 pounds. Each book weighs 1 3/4 pounds. How many books can she carry?
Notice that this model is fundamentally different from "sharing" (see below), where you split the total evenly into a given number of shares. Even though measuring and sharing are conceptually different, both use division.
"Recipes and Remainders"
Dealing with remainders with division of fraction problems...
Note: We get remainders only in "measurement" problems. When you use sharing (see below) there is never any left over.
"Sharing Pizza"
Division of fractions using the sharing model...
About Using the Number Line for a Sharing Problem
In the "sharing" model, you stretch the lower number line so the total on the upper number line is opposite the number of shares (in the pizza case, the number of people). Then you look to see where "1" is on the lower number line; this is opposite the amount that corresponds to the lower "unit."
In the pizza example, the lower line is people; we dragged 4 to 2 1/2. The answer was 5/8: how many pizzas correspond to ONE person.
In the fuel example, we dragged 5/8 to 2 1/2. The answer over the "1" was 4: how many ounces correspond to ONE mile.
Notice that the number opposite "1" on the upper number line is a unit rate.
This "sharing" setup contrasts with the "measurement" setup in the first two videos. There, we knew the unit rate (3/4 inch per marker, 1 1/2 cups of sugar per batch). So we dragged "1" to that value, and then looked to see what corresponded to the total (4 1/2 inches in the box, 5 1/2 cups of sugar).
"Width of a Field"
Division of fractions using the missing factor model...
Of course, the problem doesn’t have to be about area. Any multiplication situation becomes a division problem if you leave out a factor. Another example, using distance = rate × time:
Family G (maybe Kenny is the dad) traveled 39 miles in ¾ of an hour. How fast were they going?
Solution: 39 ÷ ¾ = 52 miles per hour.
About the Double Number-Line Model
Each of the four videos above use a "double number-line" model to show the division. This model is not required, but it might help some students better understand how division (and multiplication) work with fractions and decimals.
In the illustration below, we use "friendly" numbers. We're showing 18 (on the top) divided by 6 (on the bottom). We can see how the bottom ticks divide the interval [ 0, 18 ] into 6 equal pieces. And looking opposite the "1" on the bottom, we can see that one "share" is 3 units.
This view of the problem, this number-line picture, has other features:
It shows multiplication (e.g., 3 times 6 equals 18) as well as division.
It shows that the three different division models—measurement, sharing, and missing factor—are numerically the same.
It shows direct proportion over the whole range: we have (literally) 18 over 6 is 3 over 1. Those ratios are the same as 12 over 4, 9 over 3, and 10 over 3 1/3. Said another way, this picture shows all the equivalent fractions, and the value is whatever is over the one.
Because we can stretch the scale of either number line, we can generalize this idea to numbers that are not so friendly.
A deeper look
Think about how to show addition with two number lines. You could do addition with two rulers. Put the "0" of one ruler over the "2" of the other, and you have a machine that adds two. The illustration shows 2 + 3 = 5.
This is like the number-line model in Walking the Line. When you think about it, it works because the two rulers have the same scale. If one were inches and the other were centimeters, you couldn't use it for addition.
Our multiplication number lines work differently. They have different scales but the two zeros are always lined up.
So: for addition, you slide the zero but you keep the scale—the size of the "one"—the same.
For multiplication, you change the "one" but keep the zero the same.
It's no coincidence, then, that the identity element (oooh!) for addition is zero and the identity for multiplication is 1.
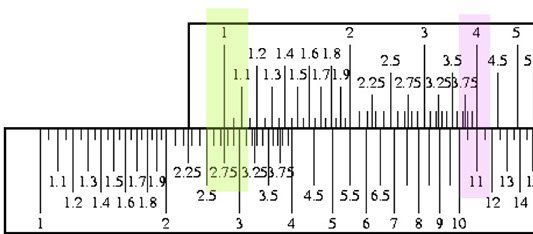
Isn't this like a slide rule?
You're old enough to remember slide rules? Slide rules used logarithms, so that you used addition in order to do multiplication. The two rules had the same scale, like addition. You slid the top rule's "1" over one factor, and read the product under the other factor.
The illustration shows 2.75 x 4 = 11.