Number Line Model for Sums and Differences
In elementary school, students often work with sets of objects to show sums and differences. For some reason, apples are often used in these word problems (have you ever wondered, why not pears?). Here is a model for the story “I have 6 apples and I give 4 away. How many are left?”
One limitation of this set model is that it is difficult to represent a quantity less than zero.
A number line can be used to represent positive and negative quantities, and the number line model can illustrate properties of signed arithmetic. The middle school standards for Number Systems call for students to use number lines in their reasoning because number lines and coordinate axes play an important role throughout middle school and secondary mathematics.
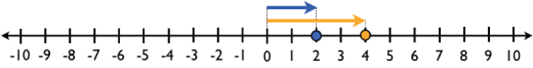
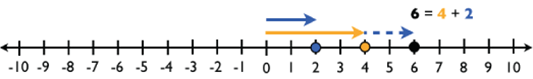
To find the sum 4 + 2 using a number line distance model, we start with a number line with 4 and 2 labeled. The arrows represent the distance from 0 to 2 and from 0 to 4.
Adding the numbers is then a matter of placing the tail of 2’s arrow at the endpoint of 4’s arrow.
The sum, 6, because 4 + 2 = 6, is where the second arrow ends.
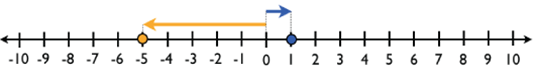
This may seem like a lot of work, but this model is especially useful for illustrating sums involving negative numbers. Since a negative number is less than 0, the arrow for a negative number points left.
Here is the number line model to show -5 + 1 = -4. First, we find the points -5 and 1 on the number line and represent them with arrows starting at 0.
Moving the tail of the arrow for 1 to the head of the arrow for -5 shows the sum:
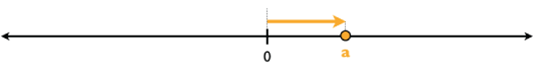
You can use a number line model to explain why a + (-a) = 0 where a represents any number. Once again, a number a is represented as a point on the number line to the right of zero (though we could choose to put a to the left of 0).
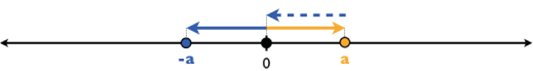
-a is the same distance from 0 as a, but in the opposite direction.
Now to find the sum a + (-a) we do the same process of moving the arrow with a length of -a until the tail lines up with the head of the arrow for a.
Notice that the sum is 0. -a is called the additive inverse of a. One key idea emphasized in 7.NS.A.1c is that subtracting is the same as adding the additive inverse.
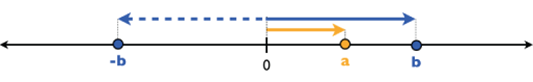
Let's illustrate the subtraction a − b on a number line. We'll start with two positive numbers a and b.
Next we look at -b, the opposite of b
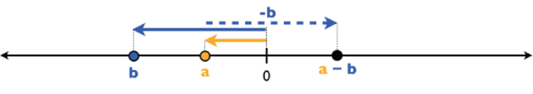
To do the subtraction, we add the opposite of b to a. That is we place the arrow with length -b so that its tail is on the head of a’s arrow.
Working with these diagrams can seem abstract. But you can quickly check the reasonableness of this answer by thinking of possible numbers for a and b. Since b > a and both b and a are greater than 0, one possible pair of numbers is a = 3 and b = 5. And, 3 − 5 = 3 + (-5) = -2. The result is less than 0, and this agrees with the diagrams above.
In the example we did above, a − b was less than zero while a and b were greater than 0. You can create similar illustrations where the sums and differences are greater than zero, less than zero, and equal to zero. Here is an example where a < 0 and b < 0, but a − b > 0