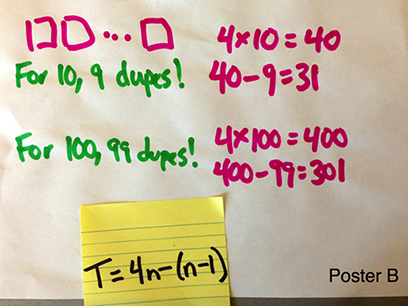Toothpick Patterns
Sixth Grade Poster Problem:Expression and Equations
This problem helps to bridge the gap in student thinking from a concrete pattern or story to a generalized, abstract arithmetic expression or formula.
Downloadable Materials
By the end of this problem, students will generate equations showing the number of toothpicks in a pattern that grows linearly. Since there are many ways to look at this problem, students might come up with equations that look different but actually result in the same values. When this situation happens, we say that the equations are equivalent. You have a vital role highlighting two important concepts: that equivalent expressions exist, and that we can simplify expressions.
Learning Objectives:
- Write, read, and evaluate expressions in which letters stand for numbers.
- Apply the properties of operations to generate equivalent expressions.
- Identify when two expressions are equivalent.
- Use variables to represent two quantities in a real-world problem that change in relationship to one another.
Common Core State Standards for Mathematics:
6.EE - 2, 3, 4, 9
1. LAUNCH
Project Slide #1, which shows three toothpick “configurations.” Each configuration has a “design number” so we know which one we’re talking about.
Ask students to look at the slide briefly and try to figure out what the pattern is—that is, how designs 1, 2, and 3 are related to each other.
As students begin to call out answers, you may want to create a table. Students will start to see patterns emerging. You can ask the following to help them along:
- How many toothpicks are in each design?
- How many squares are in each design?
- Is there a relationship between the design number and the number of squares? If so, what is it? (They’re the same.)
- Are there any other things that you notice in the toothpick pattern?
2. POSE A PROBLEM
Explain that we now want to extend the pattern to look at larger toothpick designs.
Pass out Handout #1.
Handout #1 Answer Key
It asks students to draw and analyze designs 4 and 5. The prompts on the handout are:
- Draw designs #4 and #5. How many toothpicks would you need to create the fourth and fifth design?
- Complete the table for each design.
- Do you see any patterns? If so, describe them.
Answers and likely responses:
- Design #4 has 4 squares and 13 toothpicks.
- Design #5 has 5 squares and 16 toothpicks.
Students are most likely to notice that each design has 3 more toothpicks than the previous one. Ask:
- Do you think it will always be three more, no matter how big the pattern gets?
- With this particular toothpick design, why do we end up adding three more each time we add a square?
3. WORKSHOP
Show Slide #2.
Separate students into groups. Encourage them to work together to ensure everyone is on board. Give students time to brainstorm about different ways to answer the questions and to share any patterns they may have identified in the previous problem.
Here are the prompts from the slide:
- How many toothpicks are in the tenth design?
- How many toothpicks are in the 100th design?
- How many in the nth design?
Have students make a poster that answers these questions and explains clearly how they know they are correct.
Hints you may want to offer teams as they proceed.
Look for patterns in the toothpick designs.
- How can you divide up the configuration of toothpicks to make them easier to count?
- Can you add toothpicks to make them easier to count?
Look for patterns in the numbers.
- What’s the relationship between each number and its neighbors?
Write a formula to calculate the number of toothpicks for each design. What variable(s) do you need to create the formula?
Suppose you call the total number of toothpicks T. Can you create more than one formula to calculate T? If so, create as many as possible.
The relevant variable is the number of the pattern, which is also the number of squares in the pattern. We often use n for variables like this one.
One (of many) answers is T = 3n + 1. So the tenth design will have 3(10) + 1 or 31 toothpicks; the 100th design will have 301 toothpicks.
This lesson plan provides several more solutions with detailed explanations in the next section.
4. POST, SHARE, COMMENT
Have students post their posters around the classroom.
Encourage students to travel around to view the posters created by other groups. Students should be encouraged to pose questions to other groups by attaching a small adhesive notes to posters.
During this time, the teacher should review all the posters and consider which to highlight in Phase 5.
Sample Posters
Solutions may include:
The first question (tenth design) can be answered by drawing and counting (31).
For the second question (100th design), drawing is impractical. But you could extend a table of values, or reason from it (10 needs 31 toothpicks, 20 needs 61, so 30 must be 91, etc…).
For the third—the one with n—students need to generalize. And there are many ways to do this. Some possibilities:
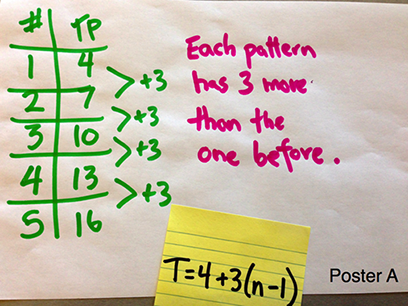
- First square plus the additions: T = 4 + 3(n – 1). (example: Poster A)
- Squares minus the duplicates: T = 4n – (n – 1). (posters B1 and B2)
- Rails and rungs: T = 2n + (n + 1). (poster C)
- The letter C, n times, and close the last: T = 3n + 1. (poster D)
- Recursive! T(1) = 4 and T(n) = T(n–1) + 3
5. STRATEGIC TEACHER-LED DISCUSSION
Facilitate a discussion of the different approaches. Select a sequence of posters to use that will help students move from their current thinking (Levels 1–3) up to Levels 4 and 5.
Level 1: Students reason solely from number patterns, and do not connect those patterns to the configurations of toothpicks. They do not generalize or make a formula, even though they can reason to a correct solution for a large number.
Level 2: Students express the “plus 3” well and abstractly, and can see where this approach comes from in the toothpicks, but cannot make a formula that works for any value of n.
Level 3: Students can make a formula that works for all values of n, but don’t connect it to the configurations of toothpicks.
Level 4: Students make a formula that works for all values of n, and can explain how each part of their formula corresponds to part of the toothpick pattern.
Level 5: Students recognize that there are different ways to construct the formula depending on how you view the toothpick configurations, and can show that these expressions are, in fact, equivalent.
Questions to ask across presentations
- Are some of the formulas easier to use than others? Why?
- Do some formulas show what is going on better than the others?
- Can you show how each part of the formula can be seen in the figure? For example, where do you see the 4 in the figure for the formula T = 4 + 3(n – 1)?
- Extension: Does your formula work for all values of n? What about 0? 1.5? (This question will raise an important point: the formula only works for positive whole number values of n. For 0, for negative numbers, and for fractions, this formula does not make sense).
Drawing the Formulas Together
Ask students, “How is it that we have different formulas for the same thing?”
- Have all students try out the formulas for a new number, such as 77.
- If all the formulas do not give the same result, figure out why, If one of them does not give the right answer (it’s 232, but make the students decide what’s right) ask the class to figure out what that means. That is, is it OK if a formula doesn’t give the right answer all the time? (It is not.)
Ask students, “Do you think the formulas give the same answer for any number?”
Ask students to use the distributive property to expand all of the expressions, and to combine like terms (i.e., “simplify”) in all of the expressions.
6. FOCUS PROBLEM: Same Concept in a New Context
If you introduce this problem in class, pass out Handout #2. Ask students to work in pairs to become familiar with the pattern in the diagram (below).
Handout #2 Answer Key
Ask students what they see in the pattern. In particular, how are the stones arranged, and what does the number of stones on each side of the garden have to do with the “garden size”?
They should see that
- the stones surround the garden.
- the height of the garden is the same as the “garden size.”
- the width of the garden is always one more than the height.
They might also notice that the number of stones along any one side is always more than the garden size because the stones are outside the garden. But they might not be able to express the problem of “overlap” or the corners clearly.
Once you’re convinced students are well-oriented to the garden context, have them work on the questions on the handout.
Answers, Approaches, and Solutions
Size 10: 46 stones.
Size 100: 406 stones.
Sides and Corners: For garden n, there there are n stones directly to the left and right. That’s 2n. Then there’s one more than that on the top and bottom, so that’s 2(n + 1). Then there are four corners, for 4 more. Total: 2n + 2(n + 1) + 4.
Fenceposts and Rails: On the left and right sides, if you go all the way to the top and bottom, you have n + 2. That’s 2(n + 2). Add the “rails” top and bottom and you get
2(n + 2) + 2(n + 1).
Recursive Approach: The first one has 10, and each one after adds four more stones. So, if S is the number of stones, then S(1) = 10, and S(n + 1) = S(n) + 4. This is correct, but hard to use for size 100! Probe to see how a student using this approach actually gets the answer. Usually students use some other way they just can’t express as a formula—until you help them see how.
Simplified formula: Any of these simplify to S = 4n + 6.
Project funding provided by The William and Flora Hewlett Foundation and S.D. Bechtel Jr. Foundation.
This work is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License.
Strategic Education Research Partnership
SERP Institute
1100 Connecticut Ave NW
Suite 1310
Washington, DC 20036
SERP Studio
2744 East 11th Street
Oakland, CA 94601
(202) 223-8555
Registered 501(c)(3). EIN: 30-0231116
©
Copyright
Strategic Education Research Partnership